Buckling

The second order effects are taken into account for the members loaded by compressive force in accordance with the chapter 5.8 of EN 1992-1-1. Following methods are avalable:

Method based on the nominal stiffness
Method based on the nominal curvature
Simplified method based on 12.6.5.2 of the standard (only plain concrete)

Slenderness criterion

The detailed analysis is performed if the slenderness is greater than limiting value λ_lim. The limiting slenderness is given by the expression 5.13N:

Where is:	*λ_lim*	The limiting slenderness
	*A, B, C*	The coefficients
	n	The relative normal force

The coefficient A is given by the expression:

Where is:

φ_ef

The effective creep ratio

The coefficient B is given by the expression:

Where is:

The mechanical reinforcement ratio

The mechanical reinforcement ratio ω is given by the expression:

Where is:	*A_s*	The total area of longitudinal reinforcement
	*f_yd*	The design yield strength of reinforcement
	*A_c*	The cross-sectional area
	*f_cd*	The design compressive strength of concrete

The coefficient C is given by the expression:

Where is:

r_m

The moment ratio

The moment ratio r_m is given by the expression:

Where is:

M₀₁, M₀₂

The first order end moments

The relative normal force n is given by the expression:

Where is:	*N_Ed*	The design value of the normal force
	*A_c*	The cross-sectional area
	*f_cd*	The design compressive strength of concrete

Method based on the nominal stiffness

This method is based on the chapter 5.8.7. The nominal stiffness is given by expression (5.21):

Where is:	*K_c*	The factor for effects of cracking, creep etc.
	*E_cd*	The design value of the modulus of elasticity of concrete
	*I_c*	The moment of inertia of concrete cross section
	*K_s*	The factor for contribution of reinforcement
	*E_s*	The design value of the modulus of elasticity of reinforcement
	*I_s*	The second moment of area of reinforcement, about the centre of area of the concrete

The factor K_c is given by expression (5.22):

Where is:	k₁	The factor which depends on concrete strength class
	k₂	The factor which depends on axial force and slenderness
	*φ_ef*	The effective creep ratio

The factor k₁ is given by expression (5.23):

Where is:

f_ck

The characteristic compressive cylinder strength of concrete at 28 days

The factor k₂ is given by expression (5.24):

Where is:	n	The relative axial force
	λ	The slenderness ratio

The buckling load based on the nominal stiffness is calculated using expression:

Where is:	*N_B*	The buckling load based on nominal stiffness
	EI	The nominal stiffness
	l₀	The effective length for buckling analysis

The total design moment, including second order moment, is calculated using formula (5.28):

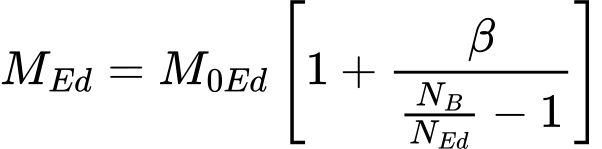
Where is:	*M_0Ed*	The first order moment
	β	The factor that depends on distribution of first and second order moments
	*N_B*	The buckling load based on nominal stiffness
	*N_Ed*	The design value of axial load

The factor β is given by expression (5.29):

Where is:

c₀

The coefficient which depends on the distribution of first order moment

The value of the factor c₀ is the input in the software. Following values are recommended according to the chapter 5.8.7.3(3):

Factor c₀	The distribution of first order moment
8.0	constant
9.6	parabolic
12	symmetric triangular

Method based on the nominal curvature

This method uses procedures given in the chapter 5.8.8. The nominal curvature is given by the expression (5.34):

Where is:	*1/r*	The curvature
	*K_r*	The correction factor depending on axial load
	*K_φ*	The factor for taking account of creep

The curvature 1/r₀ is given by following formula:

Where is:	ε₀	The strain of reinforcement at yield strength
	d	The effective depth of a cross-section

The strain of reinforcement at yield strength ε₀ is calculated using this formula:

Where is:	*f_yd*	The design yield strength of reinforcement
	*E_s*	The design value of modulus of elasticity of reinforcing steel

The effective depth of a cross-section is given by the expression (5.35):

Where is:	h	The height of a cross-section
	*i_s*	The radius of gyration of the total reinforcement area

The factor K_r is given by the expression (5.36):

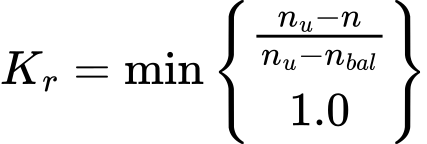
Where is:	n	The relative axial force
	*n_bal*	The value of the relative axial force at maximum moment resistance. The value 0.4 is usedin aaccordance with 5.8.8.3(3).

The relative axial force n_u is calculated using the formula:

Where is:

The mechanical reinforcement ratio

The factor K_φ is given by the expression (5.37):

Where is:	β	The factor depending on the strength class of the concrete and the slenderness ratio
	*φ_ef*	The effective creep ratio

The factor β is given by the expression:

Where is:	*f_ck*	The characteristic compressive cylinder strength of concrete at 28 days
	λ	The slenderness ratio

The nominal second order moment M₂ is given by the expression (5.33):

Where is:	*N_Ed*	The design value of axial force
	e₂	The member deflection

he deflection e₂ is calculated using formula

Where is:	*1/r*	The curvature
	l₀	The effective length for buckling analysis
	c	The factor depending on the curvature distribution

The value of the factor c is the input in the software. Following values are recommended according to the chapter 5.8.8.2(4):

Factor c	Curvature distribution
8.0	constant
10	sinusoidal

The design moment is given by the expression (5.31):

Where is:	*M_Ed*	The design moment including the second order effect
	*M_0Ed*	The design value of the first order moment
	M₂	The nominal second order moment

Simplified design method according to 12.6.5.2

This method may be used for plain concrete and lightly reinforced members according to the chapter 12.6.5.2 of EN 1992-1-1. The design axial resistance is given by the expression (12.10):

Where is:	b	The overall width of the cross-section
	*h_w*	The overall depth of the cross-section
	*f_cd*	The design compressive strength of concrete
	Φ	The factor taking into account eccentricity

The factor Φ is given by the expression (12.11):

The eccentricity e_tot is calculated using following formula:

Where is:	e₀	The first order eccentricity including, where relevant, the effects of floors (e.g. possible clamping moments transmitted to the wall from a slab) and horizontal actions
	*e_i*	The additional eccentricity covering the effects of geometrical imperfections
	l₀	The effective length for buckling analysis

GEO5

FINEC

TRUSS4

FIN EC

Online nápověda

Buckling

Slenderness criterion

Method based on the nominal stiffness

Method based on the nominal curvature

Simplified design method according to 12.6.5.2

Vyzkoušejte si zdarma náš software.
Stáhněte si aktuální Edici zdarma.

Vyzkoušejte si GEO5 - software, který šetří váš čas.

Demoverze

Vyzkoušejte si zdarma
naše programy.

Výuková videa

Podívejte se na ovládání a práci s našimi programy v praxi.

Inženýrské manuály

Stáhněte si manuály s teoretickými i praktickými ukázkami použití programů.

FIN EC

Online nápověda

Buckling

Slenderness criterion

Method based on the nominal stiffness

Method based on the nominal curvature

Simplified design method according to 12.6.5.2

Vyzkoušejte si zdarma náš software. Stáhněte si aktuální Edici zdarma.

Vyzkoušejte si GEO5 - software, který šetří váš čas.

Demoverze

Vyzkoušejte si zdarmanaše programy.

Výuková videa

Podívejte se na ovládání a práci s našimi programy v praxi.

Inženýrské manuály

Stáhněte si manuály s teoretickými i praktickými ukázkami použití programů.

Vyzkoušejte si zdarma náš software.
Stáhněte si aktuální Edici zdarma.

Vyzkoušejte si zdarma
naše programy.