0

FIN EC

Online nápověda

Stromeček
Nastavení
Program:
Jazyk:

Verification of buckling resistance of built-up cross-sections

The buckling resistance perpendicular to the strong axis is given by expression

Where is:

χ

  • The reduction factor for flexural buckling

A

  • The cross-sectional area

fy

  • The yield strength

γM1

  • The partial safety factor

The effective cross-sectional area is considered for class 4.

The slenderness λy in the direction perpendicular to the strong axis y is given by formula

Where is:

Lcr,y

  • The buckling length for buckling perpendicular to the axis y

iy

  • The radius of gyration for axis y

The relative slenderness is given by the expression

Where is:

λy

  • The slenderness in the direction perpendicular to the axis y

λ1

  • The slenderness value to determine the relative slenderness

Aeff

  • The effective cross-sectional area

A

  • The cross-sectional area

The slenderness value λ1 is given by the formula

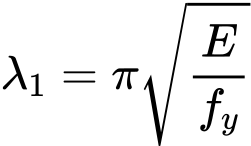
Where is:

E

  • The modulus of elasticity

fy

  • The yield strength

The value of the imperfection factor α is set according to the buckling curves a, b, c, d. The factor χy corresponds to the relative slenderness and is calculated using expression

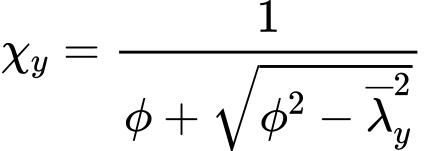
however, following condition has to be fulfilled

where

The partial cross-section fails if the specified axial force is greater than the resistance Nb,Rd,y.

The calculation of buckling resistance perpendicular to the weak axis follows. The elastic flexural buckling force Ncr is given by the expression

Where is:

lcr,z

  • The buckling length for buckling perpendicular to the axis z

kE,θ

  • The reduction factor for the slope of the linear elastic range

E

  • The modulus of elasticity

Ieff

  • The effective value of the moment of inertia, that depends on the type of connection of partial cross-sections

Following formula is used for Ieff for lacing

Where is:

h0

  • The distance of points of inertia of partial cross-sections

A

  • The cross-sectional area of partial cross-section

The second moment of area I1 is calculated for built-up cross-sections with battens using the expression

Where is:

A

  • The cross-sectional area of partial cross-section

h0

  • The distance of points of inertia of partial cross-sections

Iz

  • The second moment of area of partial cross-section

The radius of gyration i0 is given by the expression

For the slenderness

the factor μ is selected. The effective value of the moment of inertia Ieff is given by the expression

The partial cross-section fails if the specified axial force is greater than the resistance Ncr.

The verification of the shear stiffness SV follows. The shear stiffness is given by the follwoing formula for battens

or

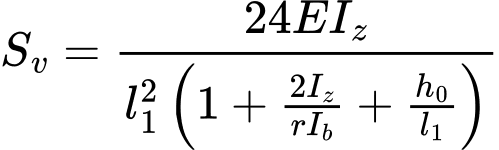
However, following expression has to be fulfilled

Where is:

l1

  • The distance of battens

r

  • The number of planes of lacings

Ib

  • The in plane second moment of area of one batten

h0

  • The distance of points of inertia of partial cross-sections

The axial force shouldn't exceed the shear stiffness SV. Also following expression has to be fulfilled

The force in the middle of the batten is calculated using formula

The force in lacing is

Where the moment MS is given by the expression

Where is:

e0

  • The bow imperfection given by the expression lcr,z/500

The buckling resistance is given by expression

Where is:

χy

  • The reduction factor for flexural buckling

A

  • The cross-sectional area

fy

  • The yield strength

γM1

  • The partial safety factor

where the factor χz corresponds to the slenderness λ, that is given by the expression

Where is:

l1

  • The distance of battens

imin

  • The minimum radius of gyration for partial cross-section

The relative slenderness is given by the formula

where

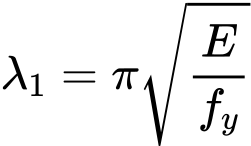
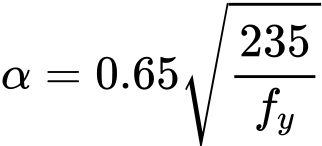
The value of the imperfection factor α is set according to the buckling curves a, b, c, d. The factor χz corresponds to the relative slenderness and is calculated using expression

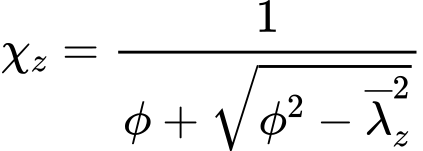
however, following condition has to be fulfilled

where

where

The shear force VS is calculated for the batten

Where the moment MS is given by the expression

Where is:

l1

  • The distance of battens

Vy

  • The entered shear force

The bending resistance of partial cross-section for bending moment My is calculated for the classes 1 and 2 according to the following formula:

The formula for the class 3:

The formula for the class 4:

Where is:

Wpl,y

  • The plastic section modulus of partial cross-section about the axis y

Wy

  • The elastic section modulus of partial cross-section about the axis y

Wy,eff

  • The effective section modulus of partial cross-section about the axis y

The bending resistance of partial cross-section for bending moment Mz is calculated for the classes 1 and 2 according to the following formula:

The formula for the class 3:

The formula for the class 4:

Where is:

Wpl,z

  • The plastic section modulus of partial cross-section about the axis z

Wz

  • The elastic section modulus of partial cross-section about the axis z

Wz,eff

  • The effective section modulus of partial cross-section about the axis z

The verification is done for two points: the mid point of the distance between two battens and in the connection of batten.

The verification in the mid point of the distance between two battens:

Where is:

n

  • The number of partial cross-sections

dN

  • The increment of axial force due to bending moment Mz

ky

  • The factor calculated according to the rules for solid cross-sections

The verification in the connection of batten:

Vyzkoušejte si zdarma náš software.
Stáhněte si aktuální Edici zdarma.

Vyzkoušejte si GEO5 - software, který šetří váš čas.

Demoverze

Vyzkoušejte si zdarma
naše programy.

Výuková videa

Podívejte se na ovládání a práci s našimi programy v praxi.

Inženýrské manuály

Stáhněte si manuály s teoretickými i praktickými ukázkami použití programů.