Active Earth Pressure - The Mazindrani Theory (Rankine)
Active earth pressure is given by the following formula:
![]()
where: | σz | - | vertical geostatic stress |
Ka | - | coefficient of active earth pressure due to Rankine | |
β | - | slope inclination | |
γ | - | weight of soil | |
z | - | assumed depth | |
| - | coefficient of active earth pressure due to Mazindrani |
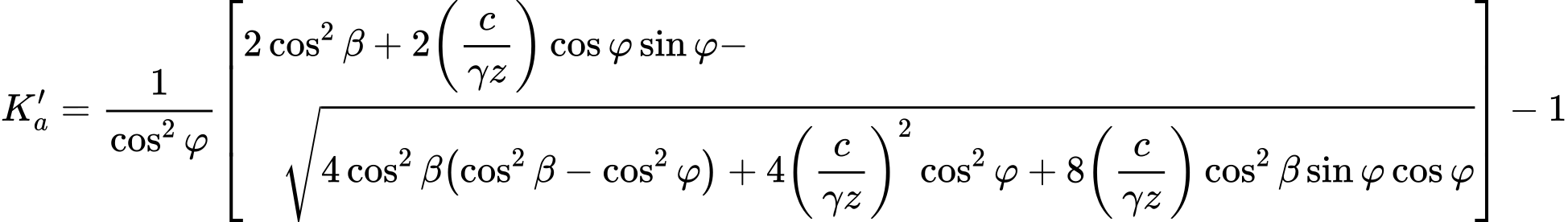
where: | β | - | slope inclination |
φ | - | angle of internal friction of soil | |
c | - | cohesion of soil |
Assuming cohesionless soils (c = 0) and horizontal ground surface (β = 0) yields the Rankine solution, for which the active earth pressure is provided by:
![]()
and the coefficient of active earth pressure becomes:
![]()
where: | φ | - | angle of internal friction of soil |
Horizontal and vertical components of the active earth pressure become:
![]()
![]()
where: | σa | - | active earth pressure |
δ | - | ||
α | - | back face inclination of the structure |
Literature:
Mazindrani, Z.H., and Ganjali, M.H. 1997. Lateral earth pressure problem of cohesive backfill with inclined surface. Journal of Geotechnical and Geoenvironmental Engineering, ASCE, 123(2): 110-112.