Analysis According to the Schmertmann Theory
Settlement of spread footing according to Schmertmann theory is based on the formula:
![]()
Where: | s | - | settlement of spread footing |
C1 | - | correction factor for footing depth | |
C2 | - | correction factor for creep settlement | |
σol | - | stress in the footing bottom | |
Izi | - | strain influence factor at the center of the ith sublayer | |
hi | - | thickness of the ith sublayer | |
Edef,i | - | deformation modulus in the ith sublayer |
Correction factor for footing depth C1 is determined as follows:
![]()
Where: | σor | - | geostatic stress in the footing bottom |
Correction factor for creep settlement C2 is determined as follows:
![]()
Where: | tyr | - | time in years after the application of load |
Diagram of the strain influence factor Iz depends on the ratio of footing length L and footing width B. The Iz is read on the horizontal axis of the graph, which is automatically generated for the task. The depth below the footing bottom is plotted on the vertical axis.
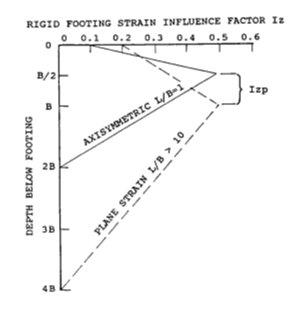 Determination of Iz factor (Schmertmann, 1978)
Determination of Iz factor (Schmertmann, 1978)
The value of Iz at the footing bottom (depth h0) is calculated as follows:
![]()
The maximum value of factor Iz(m) is determined as follows:
![]()
Where: | σzp | - | geostatic stress in the depth h1 |
The depth h1, where the factor Iz reaches its maximum value, is determined by the formula:
![]()
The maximum depth hmax, up to which settlement is considered, is calculated as:
![]()
Literature:
Schmertmann, J.H. (1970). Static cone to compute static settlement over sand. ASCE Journal of SoilMechanics & Foundations Division, 96 (3), 1011-1043
Braja M. Das (2011). Principles of Foundation Engineering (Seventh Edition)