Invariant stress and strain measures
Invariant stress and strain measures allow us to describe a general three dimensional state of stress using scalar quantities, invariants. Invariant is a quantity which does not depend on the selection of the coordinate system. It can therefore be expressed using both the variables defined in the basic cartesian coordinate system and variables associated with the principal coordinate system adopting principal stresses and strains. Point out that principal stresses can also be written in terms of invariants. Apart from allowing for a simple graphical representation of three dimensional stress state these variables are exploited in the formulation of most yield surfaces.
A short list of basic invariant stress and strain measures for the case of triaxial compression defined in terms of principal stresses and strains is provided next for illustration. Further details can be found in the theoretical manual.
- Mean stress
![]()
- Volumetric strain
![]()
- Equivalent deviatoric stress
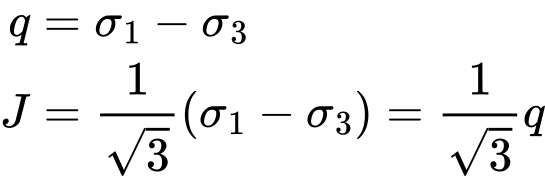
- Equivalent deviatoric strain
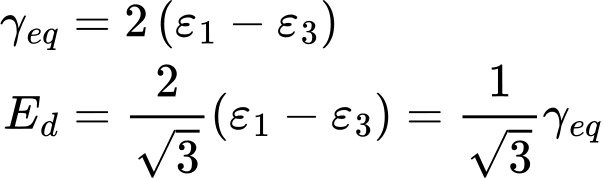
- Lodeův úhel vyjádříme obecně (pro triaxiálovou kompresi platí σ2 - σ3 = σ1 - σ3
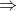 θ = 30°)
θ = 30°)
![]()